- publication
- SIGGRAPH 2022 (journal paper)
- authors
- Astrid Bunge, Philipp Herholz, Olga Sorkine-Hornung, Mario Botsch, Michael Kazhdan
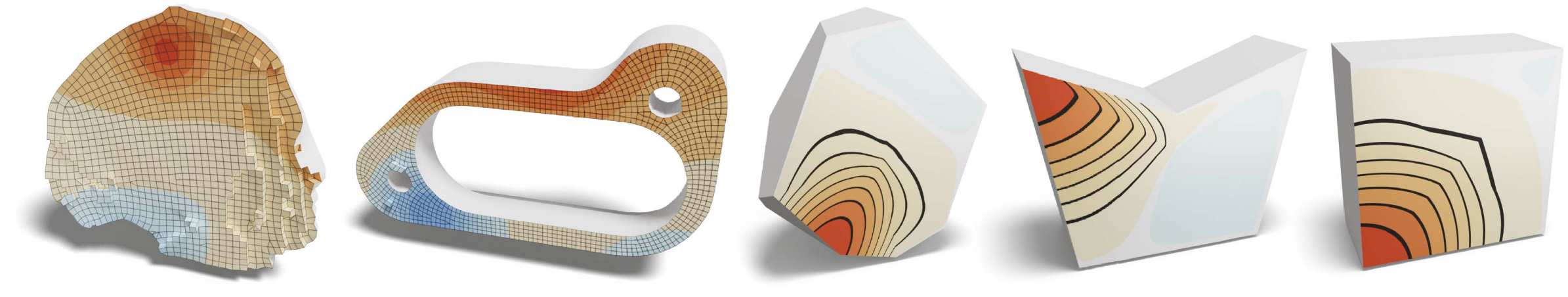
Cross-sections through the solutions to a Poisson problem on polyhedral hex-dominant meshes (left). Shape functions for irregular convex and concave polyhedra as well as for a cube (right). The shape functions are variationally optimized with respect to a smoothness criterion, leading to piecewise quadratic local bases that are almost C1 within elements. The C1 discontinuities appear as non-smooth iso-lines in the plots. Red colors indicate positive values and blue colors indicate negative values.
abstract
Solving partial differential equations (PDEs) on geometric domains is an important component of computer graphics, geometry processing, and many other fields. Typically, the given discrete mesh is the geometric representation and should not be altered for simulation purposes. Hence, accurately solving PDEs on general meshes is a central goal and has been considered for various differential operators over the last years. While it is known that using higher-order basis functions on simplicial meshes can substantially improve accuracy and convergence, extending these benefits to general surface or volume tessellations in an efficient fashion remains an open problem. Our work proposes variationally optimized piecewise quadratic shape functions for polygons and polyhedra, which generalize quadratic P2 elements, exactly reproduce them on simplices, and inherit their beneficial numerical properties. To mitigate the associated cost of increased computation time, particularly for volumetric meshes, we introduce a custom two-level multigrid solver which significantly improves computational performance.
downloads
acknowledgments
This work was supported by the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme (ERC Consolidator Grant agreement No. 101003104, MYCLOTH).