- publication
- ACM SIGGRAPH ASIA 2014
- authors
- Mario Deuss, Daniele Panozzo, Emily Whiting, Yang Liu, Philippe Block, Olga Sorkine-Hornung, Mark Pauly
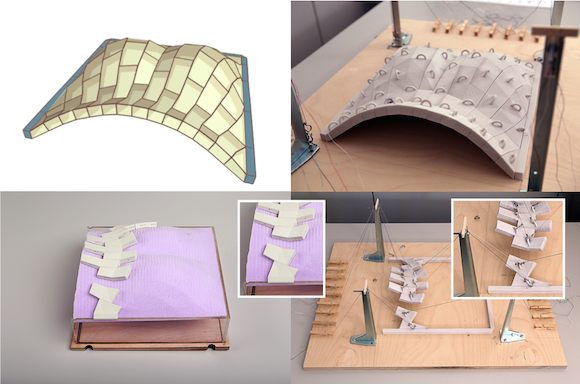
We propose a construction method for self-supporting structures that uses chains, instead of a dense formwork, to support the blocks during the intermediate construction stages. Our algorithm finds a work-minimizing sequence that guides the construction of the structure, indicating which chains are necessary to guarantee stability at each step. Top left: a self-supporting structure; Bottom: an intermediate construction stage with dense formwork and an intermediate construction stage with our method; Top right: the assembled model.
abstract
Self-supporting structures are prominent in historical and contemporary architecture due to advantageous structural properties and efficient use of material. Computer graphics research has recently contributed new design tools that allow creating and interactively exploring self-supporting freeform designs. However, the physical construction of such freeform structures remains challenging, even on small scales. Current construction processes require extensive formwork during assembly, which quickly leads to prohibitively high construction costs for realizations on a building scale. This greatly limits the practical impact of the existing freeform design tools. We propose to replace the commonly used dense formwork with a sparse set of temporary chains. Our method enables gradual construction of the masonry model in stable sections and drastically reduces the material requirements and construction costs. We analyze the input using a variational method to find stable sections, and devise a computationally tractable divide-and-conquer strategy for the combinatorial problem of finding an optimal construction sequence. We validate our method on 3D printed models, demonstrate an application to the restoration of historical models, and create designs of recreational, collaborative self-supporting puzzles.
downloads
- Paper (ACM SIGGRAPH ASIA 2014, official version available at http://portal.acm.org/)
- BibTex entry
- Video
- Additional videos (video footage for the figures in the paper)
accompanying video
acknowledgments
We thank: Hao Pan and Xiaoming Fu for providing their source code and support. Etienne Vouga, Fernando de Goes, Ramon Weber and Matthias Rippmann for providing datasets. Bailin Deng, Andrea Tagliasacchi and Sofien Bouaziz for inspiring discussions. This research was supported in part by the SNF Grant (200021-137626) and received funding from the European Research Council under the European Union’s 7th Framework Programme (FP/2007-2013)/ERC Grant Agreement 257453, ERC Starting Grant COSYM and ERC Starting Grant iModel (StG-2012-306877).